The crazy AP exam week in May (actually 2 weeks) is over, and after these two exciting weeks, all AP courses in the school have also been declared over. In the second half of the semester, the physics class assigned each of us a project assignment, and we completed related research in groups of our own choice.
After a period of careful consideration, and based on my pure love for football, I chose the topic of analyzing the principles behind "Knuckleball", finally entitled "Mechanical Analysis on Knuckleball in
Football".
From the beginning, this research topic was full of difficulties. It took a long time to build the general research framework. I had no idea about Knuckle Effect and I didn't know why it came from. After searching for relevant literature online, I found that there were papers describing related problems, but they were all mathematical models built on the basis of experimental analysis. So CFD technology came to my mind.
Computational Fluid Dynamics
As a fan of racing and airplanes, CFD technology is the most direct way to analyze such difficult problems, because it can simulate the movement of fluids by dividing the grid and specifically solve the situation in which Knuckleball is in.
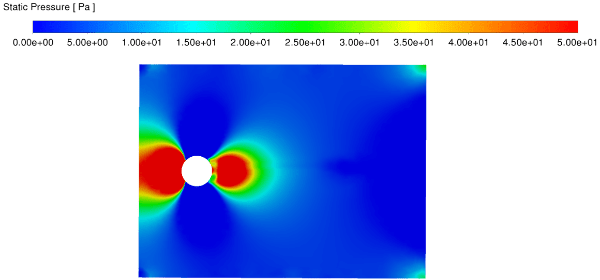
(This figure is only an example of what is CFD?)
But a headache came. After learning the relevant knowledge and downloading Ansys Fluent software and even building a 3D model of the football, I didn't know what to do next. After consulting various interpersonal relationships around me, I found a college friend who could use Ansys Fluent to help me analyze the streamlines of the airflow when the football flies in the airflow.

At the same time, I started writing the rest of the model.
After some research in related fields (because my knowledge in related fields is really lacking), my attention first shifted to Navier-Stokes equations, hoping to solve the problem from this perspective. After a series of complex calculations and simplifications, and the application of boundary conditions and the introduction of Potential Flow, I came up with a theorem like this:
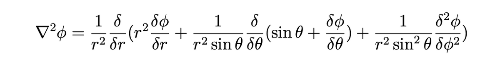
On this basis, Python was used to draw the streamline diagram simulated on the sphere. As shown below:
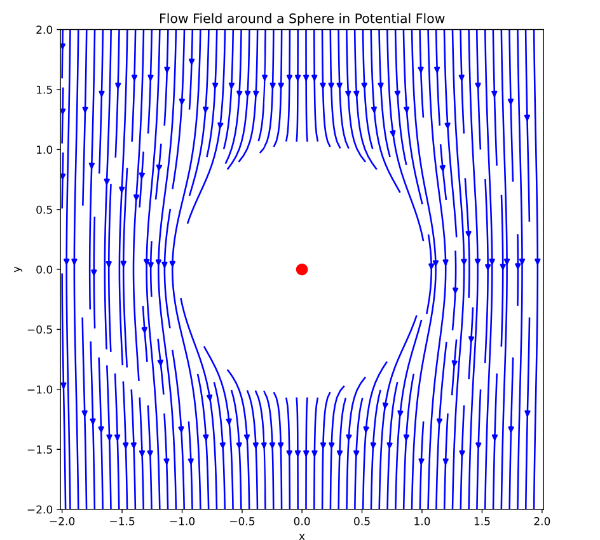
Later, based on the research of my predecessors, I completed a purely mechanical analysis, that is, analyzing the drag disaster through the law of change of the drag coefficient with the Reynolds number, and tried to explain the "underlying causes" behind the drag disaster.
This project is not just a research topic on the surface, but also brought me a lot of gains and insights. First of all, in terms of resource acquisition, I must take the initiative to communicate with my supervisor or people who are willing to help, and promote the implementation of relevant parts. In addition, I once again experienced the difficulty of scientific research, because when I delve into anything that seems simple, the profundity behind it always shocks me. In this experience, concepts such as Navier-Stokes equations or potential flow are concepts that I have never heard of before in-depth research. I think if I don’t do this project, I will hardly have the opportunity to understand this knowledge. This makes me have a higher level of awe for science and physics. I hope that I can continue to delve into the academic fields of physics and engineering in depth and maintain my interest in the future, and continue to explore new knowledge.
Wenn ich einen Schritt weiter tue, so kann mich nichts von meinem Weg abbringen.
----Ludwig van Beethoven
(Interpreted as: If I take one more step, nothing can divert me from my path.)
The End
